Inférence causale
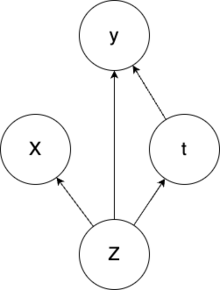
L'inférence causale est le processus par lequel on peut établir une relation de causalité entre un élément et ses effets.
C'est un champ de recherche à la croisée des statistiques, de l'économétrie, de l'épidémiologie, de la méthodologie politique et de l'intelligence artificielle.
Histoire
En 1920, Sewall Wright développe la première path analysis. Cette analyse graphique des relations de causalité entre les variables constitue selon Judea Pearl un travail pionnier dans l'inférence causale[1].
Dans les années 1970, Donald Rubin (Rubin 1974) développe un modèle causal dit modèle à résultat potentiel dans un article intitulé Estimating Causal Effects of Treatments in Randomized and Nonrandomized Studies. Ce modèle est connu sous le nom de modèle causal de Neyman-Rubin[2],[3].
Méthodes
Bibliographie
- (en) Donald Rubin, « Estimating Causal Effects of Treatments in Randomized and Nonrandomized Studies », Journal of Educational Psychology, vol. 66, no 5, , p. 688–701
- (en) Judea Pearl, « Causal inference in statistics: An overview », Statistics Surveys, vol. 3, , p. 96–146 (DOI 10.1214/09-SS057, lire en ligne)
- (en) Paul W Holland, « Statistics and causal inference », Journal of the American Statistical Association, vol. 81, no 396, , p. 945-960
- (en) Judea Pearl, Causality, Cambridge University Press,
- (en) MA Hernán et JM Robins, Causal Inference: What If, Barnsley, Boca Raton: Chapman & Hall/CRC, (lire en ligne)
- (en) Judea Pearl et Dana Mackenzie, The Book of Why : The New Science of Cause and Effect, Penguin Books, (1re éd. 2018), 432 p. (ISBN 978-0-1419-8241-0 et 0-1419-8241-1)
- (en) Scott Cunningham, Causal Inference : The Mixtape, Yale University Press, , 352 p. (ISBN 9780300251685 et 0300251688)
Revues
- Journal of Causal Inference
Notes et références
- Pearl et Mackenzie 2019.
- (en) Paul W. Holland, « Statistics and causal inference », Journal of the American Statistical Association, vol. 81, no 396, , p. 945-960.
- (en) Jasjeet Sekhon, « The Neyman— Rubin Model of Causal Inference and Estimation Via Matching Methods », dans The Oxford Handbook of Political Methodology, (DOI 10.1093/oxfordhb/9780199286546.003.0011).
